Trees

A tree is a data structure that consists of a node and a collection of nodes in a parent-child relationship.

List	Tree
Linear. Everything is is single path.	Have multiple paths. Is not linear.

There are many types of trees. We will focus on binary trees and binary search trees.

Terminology

Root - The top node in a tree. The root node is the first node in the tree.
Child -A node directly connected to another node when moving away from the Root.
Parent - The converse notion of a child.
Siblings - A group of nodes with the same parent.
Leaf - A node with no children.
Edge - The connection between one node and another.

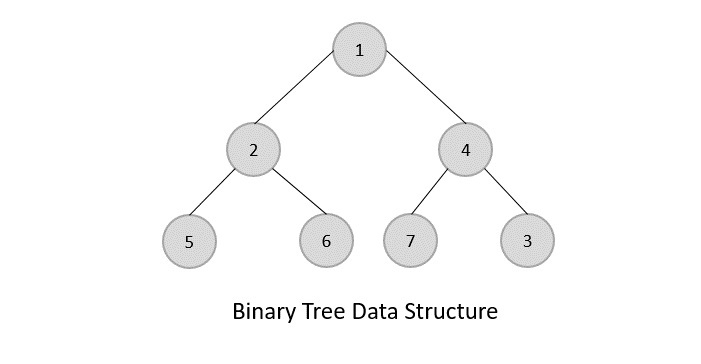
Terminology

Uses Cases For Trees

Uses Case	Description
HTML DOM	A tree that represents the structure of an HTML document.
Folders in Operating Systems	Have a hierarchy of folders.
AI	Can have a tree which describes all the possible outcomes of a game.

Binary Trees

each node can have at most, 2 children

BinaryTree

Binary Search Trees (BST)

Is a special type of binary tree.
Has either 0, 1, or 2 children.
Are sorted in a particular way in which they can be compared, sortable.
Every node to the left of a parent node is always less than the parent
Every node to the right of a parent node is always greater than the parent.

BinarySearchTree

Searching a binary search tree is done by...

if val > node: go left
if val < node: go right

Binary Search Tree (BST) Node

class Node {
  constructor(value) {
    this.value = value
    this.left = null
    this.right = null
  }
}

Binary Search Tree (BST) Class

class BinarySearchTree {
  constructor() {
    this.root = null
  }
}

Can construct a very basic BST:

const tree = new BinarySearchTree()
tree.root = new Node(10)
tree.root.right = new Node(15)
tree.root.left = new Node(7)
tree.root.left.right = new Node(9)

console.log(tree)
// Node { value: 10,
//      left: Node { value: 7, left: null, right: [Object] },
//      right: Node { value: 15, left: null, right: null } }

BST Insert()

Should be able to call insert(value) on a BST and it will insert the value into the tree in the correct spot. Recall, the left side of the tree is always less than the parent node. The right side of the tree is always greater than the parent node.

Pseudocode:

Create a new node.
Starting at the root.
- Check if there is a root, if not - the root now becomes that new node!
- If there is a root, check if the value of the new node is greater than or less than the value of the root. Easiest to use a while() loop.
  - If it is less.
    - Check to see if there is a node to the left.
      - If there is, move to that node and repeat these steps.
      - If there is not, add that node as the left property.
  - If it is greater.
    - Check to see if there is a node to the right.
      - If there is, move to that node and repeat these steps.
      - If there is not, add that node as the right property.

class Node {
  constructor(value) {
    this.value = value
    this.left = null
    this.right = null
  }
}

class BinarySearchTree {
  constructor() {
    this.root = null
  }

  insert(value) {
    var newNode = new Node(value)
    if (this.root === null) {
      this.root = newNode
      return this
    }
    var current = this.root
    while (true) {
      if (value === current.value) return undefined
      if (value < current.value) {
        if (current.left === null) {
          current.left = newNode
          return this
        }
        current = current.left
      } else {
        if (current.right === null) {
          current.right = newNode
          return this
        }
        current = current.right
      }
    }
  }
}

const tree = new BinarySearchTree()
tree.insert(10)
tree.insert(6)
tree.insert(15)
tree.insert(3)
tree.insert(8)
tree.insert(20)

//       10
//    6      15
//  3    8     20

BST Find()

Searching a binary tree to see if a particular value is within the tree.

Pseudocode:

Check if there is a root, if not - return null.
- If there is a root, check if the value of the new node is the value we are looking for. If we found it, we're done!.
- If not, check to see if the value is greater than or less than the value of the root.
  - If it is greater...
    - Check to see if there is a node to the right.
      - If there is, move to that node and repeat these steps.
      - If there is not, we're done searching!.
  - If it is less...
    - Check to see if there is a node to the left.
      - If there is, move to that node and repeat these steps.
      - If there is not, we're done searching!.

find(value) {
  if (!this.root) return console.log('no root, dummy');

  let current = this.root;
  let found = false;
  while (current && !found) {
    if (value < current.value) current = current.left;
    if (value > current.value) current = current.right;
    else found = true;
  }
  if (!found) return false;
  return current;
}

const tree = new BinarySearchTree();
tree.insert(10);
tree.insert(6);
tree.insert(15);
tree.insert(3);
tree.insert(8);
tree.insert(20);
console.log(tree.find(10)); // 10
console.log(tree.find(100)); // false

//       10
//    6      15
//  3    8     20

BST Contains()

Returns a boolean if the value is in the tree.

contains(value) {
  if (this.root === null) return false;
  let current = this.root,
    found = false;
  while (current && !found) {
    if (value < current.value) {
      current = current.left;
    } else if (value > current.value) {
      current = current.right;
    } else {
      return true;
    }
  }
  return false;
}

const tree = new BinarySearchTree();
tree.insert(10);
tree.insert(6);
tree.insert(15);
tree.insert(3);
tree.insert(8);
tree.insert(20);
console.log(tree.contains(10)); // true
console.log(tree.contains(100)); // false

//       10
//    6      15
//  3    8     20

Big O of BST

Operation	Time Complexity
Insertion	O(log n)
Searching	O(log n)

big o

Complete Code for BST

class Node {
  constructor(value) {
    this.value = value
    this.left = null
    this.right = null
  }
}

class BinarySearchTree {
  constructor() {
    this.root = null
  }

  insert(value) {
    var newNode = new Node(value)
    if (this.root === null) {
      this.root = newNode
      return this
    }
    var current = this.root
    while (true) {
      if (value === current.value) return undefined
      if (value < current.value) {
        if (current.left === null) {
          current.left = newNode
          return this
        }
        current = current.left
      } else {
        if (current.right === null) {
          current.right = newNode
          return this
        }
        current = current.right
      }
    }
  }

  find(value) {
    if (!this.root) return console.log('no root, dummy')

    let current = this.root
    let found = false
    while (current && !found) {
      if (value < current.value) current = current.left
      if (value > current.value) current = current.right
      else found = true
    }
    if (!found) return false
    return current
  }

  contains(value) {
    if (this.root === null) return false
    let current = this.root,
      found = false
    while (current && !found) {
      if (value < current.value) {
        current = current.left
      } else if (value > current.value) {
        current = current.right
      } else {
        return true
      }
    }
    return false
  }

  BFS() {
    let visited = []
    let queue = []
    let node = this.root
    queue.push(node)
    while (queue.length) {
      // while there's something in our queue
      node = queue.shift() // assign node to the first item in the queue
      visited.push(node.value)
      if (node.left) queue.push(node.left)
      if (node.right) queue.push(node.right)
    }
    return visited
  }

  DFSPreOrder() {
    let data = []
    function traverse(node) {
      data.push(node.value)
      if (node.left) traverse(node.left)
      if (node.right) traverse(node.right)
    }
    traverse(this.root)
    return data
  }

  DFSPostOrder() {
    let data = []
    function traverse(node) {
      if (node.left) traverse(node.left)
      if (node.right) traverse(node.right)
      data.push(node.value)
    }
    traverse(this.root)
    return data
  }
  DFSInOrder() {
    let data = []
    function traverse(node) {
      if (node.left) traverse(node.left)
      data.push(node.value)
      if (node.right) traverse(node.right)
    }
    traverse(this.root)
    return data
  }
}

const tree = new BinarySearchTree()
tree.insert(10)
tree.insert(6)
tree.insert(15)
tree.insert(3)
tree.insert(8)
tree.insert(20)

// console.log(tree.root.value);
// console.log(tree.find(10));
// console.log(tree.BFS());
// console.log(tree.DFSPreOrder());
// console.log(tree.DFSPostOrder());
// console.log(tree.DFSInOrder());